Venturi meters are flow measurement instruments which use a converging section of pipe to give an increase in the flow velocity and a corresponding pressure drop from which the flowrate can be deduced. They have been in common use for many years, especially in the water supply industry.
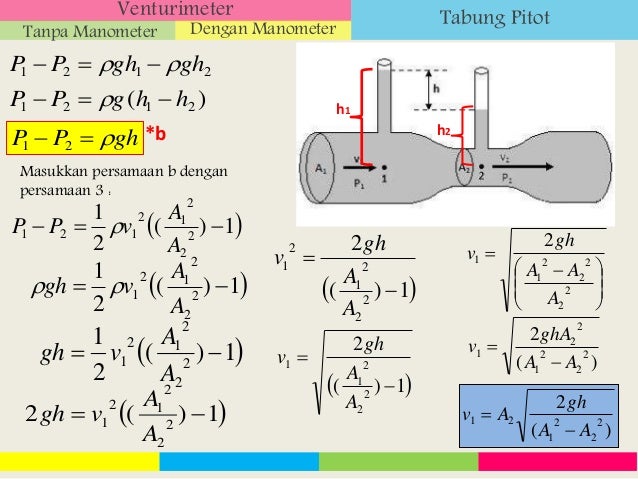
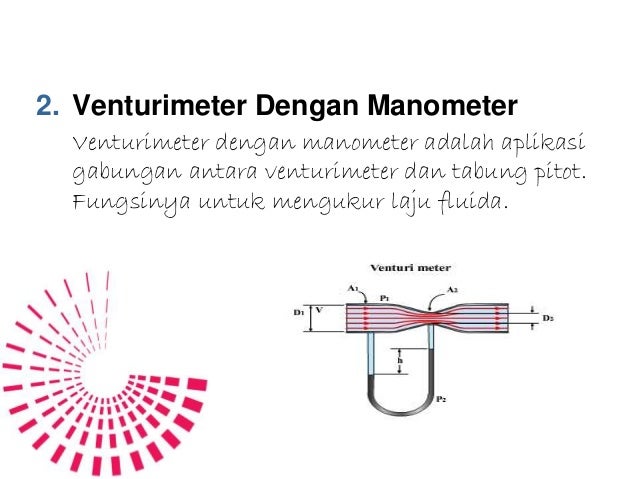
Rumus Venturimeter Dengan Manometer 13. Kegunaan dan Aplikasi Penerapan efek venturi dapat diamati pada: Cerobong Asap Dalam industri digunakan untuk mengukur laju aliran bahan kimia melalui pipa. Digunakan dalam pesawat untuk mengukur kecepatan pesawat. Karena terdapat perbedaan luas penampang pipa, maka ketika melewati A2 kecepatan aliran fulida akan lebih kecil. Terdapat dua macam tabung venturi, yaitu dengan manometeer dan tanpa manometer. Tabung venturi dengan manometer. Kedua bagian pipa (A 1 dengan A 2) dihubungkan dengan sebuah manometer zat cair yang diisi dengan air raksa. Venturi meter tanpa manometer berdasarkan prinsip Bernoulli Written By Unknown on Thursday, July 22, 2010 3:33 PM. Gunakan rumus di atas untuk menghitung laju. Manometer merkuri (ρ = 13.600 kg/m 3) dihubungkan ke saluran udara untuk mengukur tekanan di dalamnya. Perbedaan ketinggian manometer adalah 15 mm, dan tekanan atmosfir 100 kPa. Tentukan tekanan absolut di salurannya! Jawab: Diketahui: ρ raksa = 13600 kg.m-3; h = 15 mm = 5 x 10-2 m dan P atm = 1,05 x 10 5 Pa, maka P udara = P atm + ρgh.
The classical Venturi meter, whose use is described in ISO 5167-1: 1991, has the form shown in Figure 1
Figure 1. Classical Vetituri meter design. (From B. S. 7405 (1991) Fig. 3.1.4, with permission of B.S.I.)
For incompressible flow if the Bernoulli Equation is applied between two planes of the tappings,
where p, ρ and ū are the pressure, density and mean velocity and the subscripts 1 and 2 refer to the upstream and downstream (throat) tapping planes.
From continuity
whereis the volumetric flowrate and D and d the pipe and throat diameters.
Combining Eqs. (1) and (2)
Rumus Pipa Venturi Tanpa Manometer
where β is the diameter ratio, d/D. In reality, there is a small loss of total pressure, and the equation is multiplied by the discharge coefficient, C, to take this into account:
where Δp is the differential pressure (≡p1 − p2). The discharge coefficient of a Venturi meter is typically 0.985, but may be even higher if the convergent section is machined. Discharge coefficients for uncalibrated Venturi meters, together with corresponding uncertainties, are given in ISO 5167-1: 1991.
If the fluid being metered is compressible, there will be a change in density when the pressure changes from p1 to p2 on passing through the contraction. As the pressure changes quickly, it is assumed that no heat transfer occurs and because no work is done by or on the fluid, the expansion is isentropic. The expansion is almost entirely longitudinal and an expansibility factor, ε, can be calculated assuming one-dimensional flow of an ideal gas:
where τ is the pressure ratio, p2/p1, and κ the isentropic exponent. The expansibility factor is applied to the flow equation in the same way as the discharge coefficient.

Various forms of construction of a Venturi meter are employed, depending on size, but all are considerably more expensive than the orifice plate. However, because most of the differential pressure is recovered by means of the divergent outlet section, the Venturi causes less overall pressure loss in a system and thus saves energy: the overall pressure loss is generally between 5 and 20 per cent of the measured differential pressure. The Venturi meter has an advantage over the orifice plate in that it does not have a sharp edge which can become rounded; however, the Venturi meter is more susceptible to errors due to burrs or deposits round the downstream (throat) tapping.
The lengths of straight pipe required upstream and downstream of a Venturi meter for accurate flow measurement are given in ISO 5167-1: 1991. These are shorter than those required for an orifice plate by a factor which can be as large as 9. However, Kochen et al. show that the minimum straight lengths between a single upstream 90° bend and a Venturi meter in the Standard are too short by a factor of about 3.
REFERENCES
Rumus Pipa Venturi Tanpa Manometer
British Standards Institution (1991) Guide to selection and application of flowmeters for the measurement of fluid flow in closed conduits, BS 7405.
International Organization for Standardization, Measurement of fluid flow by means of pressure differential devices—Part 1: Orifice plates, nozzles and Venturi tubes inserted in circular cross-section conduits running full. ISO 5167-1: 1991.

Kochen, G., Smith, D. J. M., and Umbach, A. (1989) Installation effects on Venturi tube flowmeters, Intech Engineers Notebook, October 1989,41-43.
References
- British Standards Institution (1991) Guide to selection and application of flowmeters for the measurement of fluid flow in closed conduits, BS 7405.
- International Organization for Standardization, Measurement of fluid flow by means of pressure differential devices—Part 1: Orifice plates, nozzles and Venturi tubes inserted in circular cross-section conduits running full. ISO 5167-1: 1991.
- Kochen, G., Smith, D. J. M., and Umbach, A. (1989) Installation effects on Venturi tube flowmeters, Intech Engineers Notebook, October 1989,41-43.
